1. Classroom Resources
  2. Algebra
  3. Olympic Climbing

Olympic Climbing

Midpoint Distance Average Rate of Change

Objective

The objective of this activity is to view a graphical representation of the Olympic Speed Climbing route and use the distance formula and the midpoint formula to answer questions. Students also explore how the rate of change varies during a race.

During the 2021 Summer Olympics in Tokyo, competition climbing became an Olympic sport for the first time. Athletes competed in three separate disciplines: speed climbing, bouldering, and lead climbing. In speed climbing, two climbers race side-by-side to scale identical routes on a 15m high wall set at an angle of 95 degrees.

During one race, Alberto Lopez from Spain competed against Adam Ondra from the Czech Republic.

Find the Midpoint

A coordinate plane has been placed over a rendering of the climbing route. The starting position for Alberto Lopez is at the point (6, 1.75) and the final position is at (5.5, 15). What are the coordinates of the midpoint between the starting and final positions?

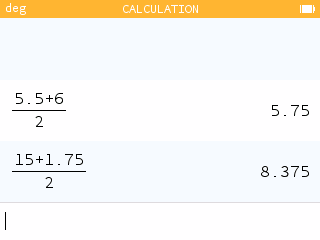
Compute the middle point on the Numorks calculator

The midpoint is at (5.75,8.375)\left ( 5.75,8.375 \right ).

The wall graph

Source: Olympics.com

Find the Distance

Alberto Lopez's path is marked and the coordinates of his handholds are listed in the table below along with the time in seconds.

  1. Between which two holds did Lopez move the farthest? Justify your answer.

    2. Students can use the image to select candidates for the largest distance and confirm using the distance formula. Candidates: 2 to 3, 6 to 7, 15 to 16.

    Candidate Distance Formula Distance
    Hold 2 to Hold 3 (5.15−5.25)2+(4.25−2.75)2\sqrt{(5.15-5.25)^2+(4.25-2.75)^2} 1.503m
    Hold 6 to Hold 7 (4.75−5.6)2+(7.75−6.3)2\sqrt{(4.75-5.6)^2+(7.75-6.3)^2} 1.681m
    Hold 15 to Hold 16 (5.5−5.25)2+(15−13.5)2\sqrt{(5.5-5.25)^2+(15-13.5)^2} 1.521m

    Lopez moved the farthest between hold 6 and hold 7. The distance was 1.681 meters.

  2. Determine the total distance traveled by Lopez.

    3. Use the distance formula to compute the distance between each hold, then sum the distances.

    Total distance = 15.215 meters

  3. Is Lopez climbing faster, slower, or the same speed as he nears the top? Justify your answer.

    4. Answers may vary. One strategy is to find the distance traveled between two holds and divide it by the change in time. Since some holds occurred at the same time, alter this strategy by finding the distance traveled between hold 1 and 3, hold 2 and 4, etc. Then divide by the change in time. With this strategy, it appears that Lopez is speeding up as he climbs!

The path on the wall
Hold Time (s) x y
1 0.0 6 1.75
2 0.7 5.25 2.75
3 1.5 5.15 4.25
4 1.6 5.5 4.75
5 2.1 5.15 5.5
6 2.5 5.6 6.3
7 3.0 4.75 7.75
8 3.4 5 8.4
9 4.1 5.15 9.5
10 4.3 4.7 10.2
11 4.8 5 11
12 4.8 5.5 11.25
13 5.4 5.5 12.5
14 5.9 6 13
15 5.9 5.25 13.5
16 6.56 5.5 15
For more information on Olympic Sport Climbing: